Riddle 3

For this riddle, I need you to use the following to create a true mathematical equation.

2  3  4  5  +  =

You may only use each of those once, and it must be true. I am sure some of you are thinking, well what about:

2 + 5 = 3 + 4

But we cannot do this, because we are only allowed to use "+" once. You may combine the numbers, so take the "2" and the "3" and make "23". 

Again, it is a fun riddle, so try and solve it yourself. Do not Google it, have fun with it. 

I will be publishing the answer on Thursday. If you come up with a solution to this before then, keep it to yourself and let me know on Thursday. 

Answer to Riddle 2

The answer to the second riddle is as follows:

I would put my straight line on the '+' sign, making it a 4. This gives me a sum of 545 + 5 = 550, which is true. 

Again, congratulation to Ebony for solving this one. 

Volume of 3D shapes

Today we had a look at calculating the volume of 3D shapes. The general formula for finding the volume of a shape is:

Volume = Area of a Face x Depth (or Height)
or more generally, V = A x D

Now we know how to calculate the area of basic shapes, using the formulas in this table:

We can use these formulas to calculate the area of the face of our 3D shape. When we pick which face to calculate, we need to pick the face that has a constant profile throughout the shape. We need to pick the face so that if we were to cut the shape and take a cross section, the face would be the same dimensions, no matter where we cut it.

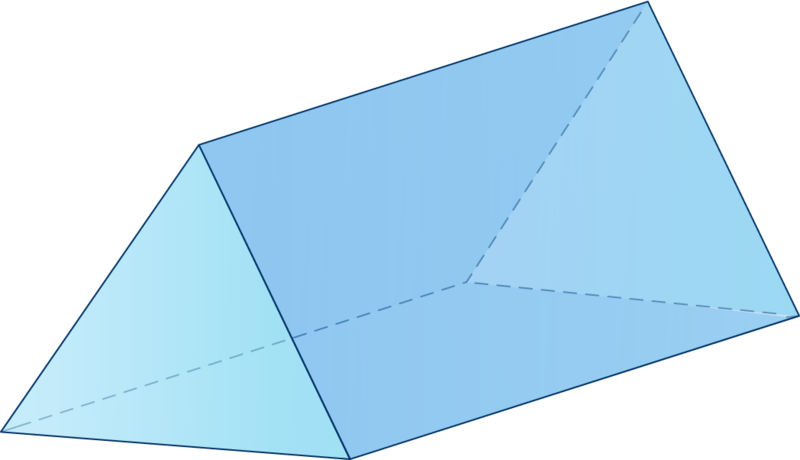
For example, take the following prism.

If we wanted to find the volume of this, we would pick the triangle as our face, because at any point along this prism, we will always have that triangular cross section. We would not pick one of the rectangular faces, because they are not a constant cross section throughout the shape. 

Once we know the area of the triangular face, we simply multiply this by the depth of the prism and we will have the volume. 

Another example could be this pentagonal prism:

We would pick the pentagonal face as our face to use, since at any point along the shape it will still have this as a cross section. Again, once we have the area of this face, we can then multiply it by the depth of the prism and get the volume. 

So for example, find the volume of the following prism: 

First, we will find the area of the face of this prism. In this case, we will use the from face. This is a rectangle with dimensions of 10cm and 4 cm. The area of this is: 

A = 10cm x 4cm 

A = 40cm square

Now that we have the area of the face, we can simply multiply this by the depth of the shape. In this case it is 17cm deep, so we get: 

V = 40cm square x 17cm

V = 680 cubic centimeters. 

Our units for volume are cubed. For example we may have cubic millimeters, cubic centimeters, cubic meters, cubic kilometers, etc. These are written as mm³, cm³, m³, km³ , etc

One last important point is that generally when we talk about volume in the 'real world' we would use the units of milliliters (mL) or liters (L). To convert between our units that we calculate in (i.e. cubic centimeters) and these 'real world' units, we use the following conversion: 

So if a prism has a volume of 45 cubic centimeters, we know it also has a volume of 45mL. 

It is also important to remember that 1L is 1000mL. 

I have uploaded a worksheet into the "Worksheets" tab, so work through that. I need that finished by Thursday's lesson, because we will use Thursday's lesson as a revision lesson for a test coming up. 

As always, if you have any questions comment on this post, or email or chat to me in person. 

Riddle number 2

I have another riddle for you. For this one you need the following equation:

The riddle is: Add one straight line to this equation to make it true. 

Just one rule for this one, you cannot put a line through the equals sign, to make it "not equal". 

If you come up with an answer before Tuesday's lesson, let me know. Do not tell anyone else, let them try and solve it for themselves. 

Same as last time, do not try and Google the answer. That is not the point of these riddles. They are just meant to be a little bit of fun, so try and solve it. If you can't, don't stress. I will reveal the answer on Tuesday. 

Areas of Composite Shapes

Today we still looked at areas, however we were looking at the area of composite shapes. A composite shape is an irregular shape that is made up of 2 or more regular shapes stuck together (or cut out). For example:

This is a composite shape, because it is made of 2 regular shapes. It has a rectangle with a triangle stuck to the right hand side of it. We know how to find the area of a rectangle and we also know how to find the area of a triangle, so to find the total area of this shape, we find the areas of the 2 regular shapes and add these together. 

So working this out, the area of a rectangle is length times width, so the area of this rectangle is       12 x 14 = 168 centimeters square. The area of a triangle is (base times height) divided by 2, so the area of this triangle is (8 x 12)/2 = 48 centimeters square. So the total area of this shape would simply be the area of the triangle plus the area of the rectangle, which would be 48 + 168 = 216 centimeters square. 

We do have some more complicated shapes. For example, we may have: 

The easiest way to do this one is to break it into 3 rectangles, and find the area of these three rectangles, then add the areas together. I would break it up like so: 

Now we need to find the area of each of the rectangles. 

First we will look at rectangle 1: The are of this one will simply be 12 x 4 = 48 meters square. 

Now looking at rectangle 2: This one is a little more complicated. We are told one of the side lengths, but we don't know the other. We can work this out however. We know the whole left hand side is 12m, and we know that there is 4m above rectangle 2, and 5m below it. So this means that the total side length must be 12 - 4 - 5 = 3m. So we can now work out the area of this rectangle. It would be     5 x 3 = 15 meters square. 

Finally rectangle 3: This one is a little easier. We know the height is 12m and the width is 3m so the area will be 12 x 3 = 36 meters square. 

Now that we know all the area of all of our rectangles, we can find the area of the whole shape. This will be 48 + 15 + 36 = 99 meters square. 

You should now be able to work through the rest of the worksheet on area. Try and have all of this finished by Tuesday. Remember to do all of the questions on this sheet. 

As always, feel free to comment on here if you have any questions, or email or chat to me in person. 

Riddle Answer

The answer to the riddle involved thinking a little outside the box. If you use the number 5 three times with a plus sign you can make it equal to 60. You would do it like so:

Congratulations to Ebony for getting the answer.  Well done!

Riddle

On a side note, I did give the students in the class a riddle. If I have the following:

In essence I have 3 identical numbers (20) and a plus sign (+) and I have made it equal 60. I want you to find me a sum that uses 3 different identical numbers (so not 20) and a single plus sign (+) and make it also equal to 60. I will reveal the answer in class tomorrow, so if you can come up with a solution by then, let me know before the start of class. 

Do not try and Google the answer, that would just be cheating yourself! Have a bit of fun with it, and if you can't see an answer don't stress too much. 

There may be a prize in it for anyone who gets the answer before class on Friday 27/08/2015 (i.e. before 10am) 

Area of a Shapes

So today we had a look at finding the area of a shape. The area of a shape is the total space a shape takes up. The units we use are slightly different to the units we use for length. When we measure lengths or perimeters we are just measuring those in meters, or centimeters, or millimeters, or kilometers, or others. When we are finding areas we find them in units such as meters squared, or centimeters squared, or millimeters squared, or others. These can be written like so:

The little "2" stands for "squared". 

There is one other special unit of measurement used when finding areas. This is a hectare (often written as just "ha"). This is often used for giving the area of land. One hectare is 10000 meters squared. 

Now to actually find the area of shapes, we use special formulas depending on what shape we have. I have made a nice table for you to use which shows you how to find the areas of the shapes we need to find in this unit. It looks like this:

The three shapes we really have to know how to find areas for in this unit are rectangles, parallelograms, and triangles. Now it is important to remember that a square is a special type of rectangle, where the length and width are the same. We do use the same formula for this though (length x width) 

The table above gives us all the information we need for finding the area of the shapes we need. One last point that I need to stress is the fact that you will need to make sure your units are the same. For example, if you had a rectangle with a length of 2.7m and a width of 35cm, you would have to make sure these are both either centimeters or meters. You can do either, but if the question asks you for your answer in square meters, it would make sense to convert the 35cm into meters, so your answer is in square meters. 

I have uploaded a new worksheet (as well as answers for this worksheet) in the "Worksheets" tab. You should be able to work through all of these questions in the next few days. Remember I have cut some questions out, so do all of the questions on the sheet. 

As always, if you have any questions feel free to comment on this post, or email or chat to me in person. 

  

Perimeter

Today we had a look at calculating the perimeters of shapes. The perimeter of a shape is the total distance around the shape. So for example, if we have a shape like this, the perimeter would be found by just adding the sides together. We would get:

120m + 27.5m + 60m + 32m + 36m + 35.5m = 311m 

One other key piece of information when calculating perimeters is understanding what these dashes on the sides of shapes mean

If sides have these dashes, it means they are the same length. So any side with a single dash on it is the same length as the other sides with a single dash. So for example; 

Each side with a dash on this shape (so all of them) is 18.5cm long. 

Some shapes can have some sides with one dash, and some sides with 2 or more dashes. Only the sides with the same number of dashes are of equal length. So for example:

This shape has 2 sides with a single dash, so these would both be 140cm long. It also has 2 sides with two dashes, so these would both be 6.7m long. 

There is a perimeter worksheet in the "Worksheets" tab. Do all questions on this sheet, and check your answers as you go. I have cut any irrelevant questions out, so you should be able to get this done before Thursday the 27th August. 

As always, feel free to comment on this post if you have any questions, or email or chat to me in person. 

New unit - Measurement

Today we started a new unit on measurement. This involves firstly reminding ourselves how we convert between the units we use when measuring lengths (i.e. meters, centimeters, millimeters, kilometers). Once we have mastered this, we will go on to calculate the perimeter and area's of shapes, as well as the volume of prisms.

To start with, we will look at converting between units of length. First of all, we have to remind ourselves of the difference between each. We need to remember that:

• 1 kilometer is 1000 meters
• 1 meter is 100 centimeters
• 1 centimeter is 10 millimeters

Now to convert between each, the following diagram is really helpful:

Because there are 1000 meters in a kilometer, if we wanted to find out how many meters 3 kilometers were, we would multiply the 3 by 1000, as the diagram says. The diagram also shows us how to convert between many different units. It is important to remember that km is short for kilometers, m is short for meters, cm is short for centimeters, and mm is short for millimeters. 

I have uploaded a worksheet into the "Worksheets" tab. I have circled the questions I would like you to do. If you use this diagram, this worksheet should be quite easy to complete, so simply finish off this sheet for homework by Thursday. 

As always, feel free to comment on this post if you have any questions on this topic, or email me or chat to me in person. 

Test Reminder

Just a reminder about the test tomorrow (Friday the 13 August). This will be on percentages and decimals. You will need a calculator and a pen/pencil. You are also allowed to bring in 1 A5 information sheet, with any information or examples that you may need (a good idea would be to put how to convert between percentages, decimals and fractions on there).

If you want some last minute revision, work through the questions from the chapter review. The relevant questions are listed in one of the posts below.

As always, if you have any questions feel free to comment on this post, or email or chat to me in person.

Test revision

We are at the end of our unit on percentages and decimals. This means it is time for a test. We will be having the test on Friday the 14th of August. For revision, you have a "Chapter Review" in your booklets. Work through the following questions from this:
(Ignore the "Fill in the blanks" section, skip straight through to the questions under the heading "Fluency")

2, 3, 5, 7, 9,
11 a, c,
12 a, c,
13 a, c, e
14 a, c, e
15 a, c, g, i
16 a, c
17 a, c, e
18 a, c
19 a, c
20, 23, 24, 25, 28

These do seem like a lot of questions, however this is work for all of Tuesday's lesson, as well as Thursday's lesson, and homework for the week.


As far as the test goes, you will be allowed to bring in an information sheet. This must be A5 in size and only single sided. On this, you may include any information or examples you think you may use. You will also be able to use a calculator for part on the test, so be sure to bring one of these.

As always, if you have any questions feel free to comment on here, or email or chat to me in person.

Finishing Percentages and Decimals

We are now at the end of our unit on percentages and decimals. To finish off all the unit work, please complete the following work from section 4.8 in your booklets.
1 - a, b, d, e, g, h,
2 - a, c, e, g, i
6, 7, 9, 10
13 - a) i, iii, v
14
Extension: 17

Some of this was set for homework on Tuesday, however if you haven't finished that yet finish it as well as the extra work from 4.8 for homework before our next lesson on Tuesday.

Because we are nearing the end, we will be having a test some time next week. We will discuss this in class on Tuesday. Tuesday's lesson will be dedicated to revision, looking at the chapter review at the end of your booklet.

Working With Percentages

We see percentages every day. We may see percentages on our food labels.

We also see them in news reports when we are given statistics

We are also faced with them when we are shopping

Because we see these quite often, it is essential that we know how to work with these. 

One example that we may face could be finding a percentage of a value. For example, trying to find 10% of $820. 

To do this, or first step is to convert the percentage to a decimal. In this example, 10% becomes 0.1

We then simply multiply this decimal by our whole value. So in this example, we multiply 0.1 by $820. 

This gives us a final answer of $82. So 10% of $820 is $82. 

Another example could be finding the percentage in a group. An example of this could be to find the percentage of students with brown hair if 12 out of 30 students in a class have brown hair. 

Our first step should be to make a fraction. We know from our units on fractions that 12 out of 30 can be written as a fraction 12/30. 

We then use our skills to convert a fraction to a percentage. When we do this we get 40%. 

So we know that if 12 out of 30 students have brown hair, 40% of the class have brown hair. 

Finally, another example could be when we see an item in a store reduced by a percentage. For example, we may see a pair of jeans originally priced at $80 but are then reduced by 20%. To work their reduced price, we find what 20% of $80 is, and then subtract this from the original price. 

So first we find out what 20% of $80. To do this, we multiply 0.2 by $80 and get $16. We now know that we are reducing the jeans by $80. 

Next, we subtract the $16 from the $80 and get $64. 

We now know that jeans originally marked at $80, but then reduced by 20% would cost you $64. 

You now have the skills to move onto section 4.8 of your work booklet. The questions I need you to do are:

1: a, b, d, e, g, h

2: a, c, e, g, i

6, 7, 9

If you finish these and would like to move onto some more work, look at questions 10 as well as 13, part a, questions i, iii, and v.

As always, if you have questions please feel free to comment on here, or email me or chat to me in person.